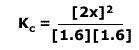|
The equilibrium law is a mathematical treatment of the ratio of product and reactant concentrations under equilibrium conditions. It is also called the law of mass action. |
|
The equilibrium expression
The concentrations of reactants and products are constant at equilibrium. This means that their ratio can be expressed as a simple mathematical formula:
| For any reaction of the form: |
 |
|
| The equilibrium expression is given by: |
 |
Where:
- [A] = the concentration of component A,
- [B] = the concentration of component B, etc.
Kc is called the equilibrium constant with respect to the concentration of the reactants and products (that's why there is a subscript 'c' following the letter traditionally used for a constant value, 'K'.
NOTE In the above example, the coefficients of the equation appear in the equilibrium expression as powers to which the concentrations of the reactants and products are raised.
|
Example: Show the equilibrium expression, with respect to concentrations, for the following reversible reaction:
Kc is given by the product concentrations raised to the power of their coefficients, divided by the reactants concentrations raised to the power of their coefficients:
|
2HI(g) 
Solids and liquids
Only those substances that appear in the equilibrium in a form that can be expressed in terms of concentration can appear in the equilibrium expression.
This means to say that solids NEVER appear and liquids only appear if they are part of a homogeneous system.
|
Example: Show the expression for the equilibrium that exists between iron, water, hydrogen and iron(III) oxide at elevated temperatures:
Inspection of the equation reveals that both iron and iron oxide are in the solid states, i.e. they do not have a concentration. This means that they cannot appear in the equilibrium expression, therefore:
|
Equilibrium calculations
All equilibrium calculations require a knowledge of the balanced equation.
Using the coefficients of the equation, the concentration of the reactants and products can be found from the equilibrium constant and vice versa.
The following methodology is used.
Equation: |
CH3COOH
|
+
|
C2H5OH
|
 |
CH3COOC2H5
|
+ |
H2O
|
|
Initial moles:
|
1
|
|
1
|
|
0
|
0
|
|
Total volume /dm3 =V |
|
|
|
|
|
|
|
|
Initial concentration/ mol dm-3
|
1/V
|
|
1/V
|
|
0
|
0
|
|
Equilibrium moles |
1-x
|
|
1-x
|
|
x
|
x
|
|
Equilibrium concentration |
(1-x)/V
|
|
(1-x)/V
|
|
x/V
|
x/V
|
The balanced equation tells us the relationship between the moles of reactants
and the moles of products. In the equation above 1 mole of each reactant produces
1 mole of each product. 1 mole of each reactant has been chosen purely for convenience.
Any other quantity could have been used in the example.
The equilibrium law requires the concentrations of all of the components of
the equilibrium to be known, therefore the total volume must also be known,
as well as the molar amounts. In fact, if the coefficients are equal on both
sides of the equation the volume terms cancel out. This is the case in the example
equation, but is not always the situation.
The number of moles of product formed at equilibrium depends on the number of
moles of reactants used up. The above table suggests that 'x' moles of reactants
are used up from an initial number of 1. This leaves (1-x) moles of each reactant.
Again, this relationship depends on the coefficients
of the equation. If two moles of a product are formed from one mole of a reactant,
then if x moles react there will be (1-x) moles of reactant remaining, whereas
there is 2x moles of product at equilibrium.
The last step is to convert the equilibrium moles of each component into concentrations
using the volume.
In the above example the equilibrium law: 
In this case, the volume cancels out from all of the concentrations and the
equilibrium law becomes: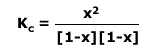 
If the value of Kc is known then the value of x can be calculated using the quadratic formula. However, use of quadratic equations is not required for IB chemistry. Calculations may be required when 'x' is given in the question.
|
Example: When 1.0 mole of ethanoic acid is mixed with 1.0 mole of ethanol and the mixture
allowed to reach equilibrium the following reaction occurs: CH3COOH(l)
+ C2H5OH
|
Use of approximation
In the equilibrium law equation various simplications may be applied by the use of approximations that do not affect the final answer, but which do make the calculations much easier.
If the value of the equilibrium constant is very small (less than 1), this means that very little of the reactants are used up in the equilibrium reaction.
We can approximation the equilibrium concentration of the reactants to the initial concentration without affecting the final answer.
|
Example: In an experiment to investigate the equilibrium: N2(g)
+ O2(g) In this case the equilibrium constant is very small, so we can assume that the equilibrium concentration of the reactants is the same as the initial concentration.
Here we have assumed that 'x' moles of reactants have reacted and hence formed 2x moles of product. As we are given concentrations not moles, we can assume that the volume is 1 dm3 for convenience. By the equilibrium law: Filling in the values: Rearranging and substituting for Kc: 4x2 = 2.56 x 1.7 x 10-3 Therefore x = √1.09 x 10-2 Therefore x = 0.104 The final concentration of NO is 2x = 0.208 mol dm-3 |
