|
The concept of half-life is important in radioactivity as it follows first order kinetics. |
|
Definition of half-life
The half-life is the amount of time taken for one half of the initial reactants to be used up. The half-life is given the symbol t1/2.
For example, if the initial concentration of a reactant is 2.0 mol dm-3, then the time taken for the concentration to reach 1.0 mol dm-3 is called the half-life. It does not have to be the concentration that is measured, providing that the quantity is directly proportional to the amount (number of moles) of the reactant.
For example, the pressure of a gas could be used as a measure that is proportional to the number of moles.
For a first order reaction, the half-life is always constant regardless of the amount of initial reactant. A typical process that follows first order kinetics is the radioactive decay of a radioisotope. It is often simpler to look at radioactive decay to understand half-lives.
A graph of the amount of substance remaining against time for a radioactive material is called a decay curve. The half life can be obtained from a decay curve by choosing two suitable points on the curve for which the y value is halved.
As the half life is a constant, then it does not matter which two points are chosen, provided that y1 = 2y2
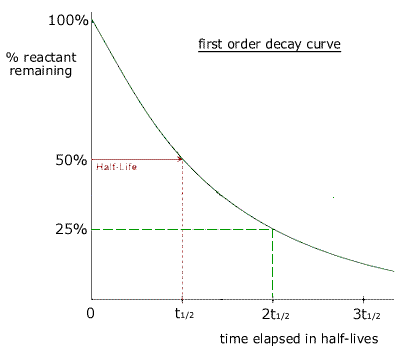 |
The time taken for the amount of reactant to be reduced by a factor of one half is always constant. In this case it is the decay of radioactive atoms that follows first order kinetics. |
The rate or decay constant
As the half life is always constant for a first order process, then the rate constant of the reaction can be related to the half life by a simple equation:
First order reaction:
| Rate = k[A]1 |
And for the half-life:
| t1/2 = ln2/k |
Where k is the rate constant and ln2 is the natural log of 2 (= 0.693).
This provides a simple means of calculating the rate of a first order reaction from the rate constant and the concentration of reactants at any particular time.
|
Example: Given that the half life of a first order reaction is 25s, calculate the rate of the reaction when the concentration of reactant is 2.0 mol dm-3. t1/2 = ln2/k Therefore: k = 0.693/t1/2 Therefore: k = 0.693/25 = 0.0277 s-1 For a first order process, Rate = k[A] Therefore when [A] = 2.0 mol dm-3 Rate = 0.0277 x 2 = 0.0554 mol dm-3 s-1 |
Note that for radioactive processes, the rate constant is called the decay constant and symbolised using the Greek letter lambda.
Non first order reactions
The half life for a reaction that is not first order is NOT constant. It is dependent on the concentration of the reactants.
The actual relationship between the half-life, the rate constant, and the concentration of a second order reaction is more complex and beyond the scope of the IB Syllabus.


