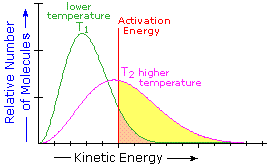
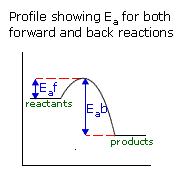
Higher-level only
The Arrhenius equation relates the rate constant, k, to the activation energy of a reaction, Ea. It affords a means of determining activation energies by experimentally finding the rate constant at different temperatures.
Syllabus ref: R2.2.12Reactivity 2.2.12 - The Arrhenius equation uses the temperature dependence of the rate constant to determine the activation energy. (HL)
- Describe the qualitative relationship between temperature and the rate constant.
- Analyse graphical representations of the Arrhenius equation, including its linear form.
Guidance
- The Arrhenius equation and its linear form are given in the data booklet.
Tools and links
The Arrhenius equation
The actual dependence of the rate constant on temperature is given by the Arrhenius equation.
|
k = Ae-Ea/RT
|
Where:
- k is the rate constant
- A is the Arrhenius factor (different for every reaction)
- e is the natural log base
- Ea is the minimum energy required for a reaction to take place (known as the activation energy)
- R is the universal gas constant (8.31 J kg-1 ºC-1)
- T is the absolute temperature in Kelvin
Arrhenius' constant, A - the pre-exponential factor
It is possible for colliding particles to possess enough energy for reaction, but still not have a successful collision (one that results in reaction). This is accounted for by the Arrhenius constant 'A' , also called the pre-exponential or frequency factor.
Imagine a collision between two cars; clearly more damage is going to be caused by a head on collision than a glancing scrape.
The Arrhenius constant (pre-exponential or frequency factor) is a number between 0 and 1, that reflects the proportion of successful collisions amongst those particles with enough energy for reaction.
For example, when A is very small, only a small proportion of collisions lead to reaction, regardless of the energy. When A = 1, all collisions with sufficient energy cause reaction.
Using the Arrhenius equation
In reality, the basic form of the Arrhenius equation is not very convenient for graphing or analysing date. To analyse experiments at different temperatures we usually use the natural log form of the equation:
k = Ae-Ea/RT
taking natural logs throughout this gives:
lnk = lnA - Ea/RT
Thus a plot of lnk against 1/RT, 1/T or any variation, will allow us to find the activation energy of a specific reaction as a function of the gradient, and the Arrhenius constant as a function of the intercept to the y axis.
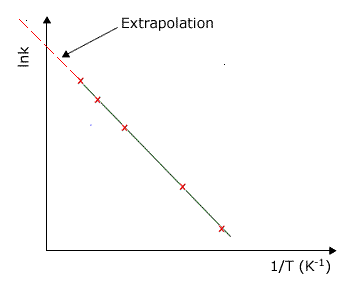 |
A typical plot used to calculate the activation energy from the Arrhenius equation. In this graph the gradient of the line is equal to -Ea/R Extrapolation of the line to the y axis gives an intercept value of lnA When the temperature is increased the term Ea/RT gets smaller. This means in turn, that the term e-Ea/RT gets bigger. |
Simultaneous equations
Alternatively two results may be analysed simultaneously to obtain values for Ea, the activation energy and the Orientation factor, A. This is not particularly reliable as only two values for the rate constant are used at two different temperatures. This can introduce large errors because of too little data.
|
Example: Calculate the rate constant when T = 300K (A = 0.3, Ea = 50kJ mol-1) k = Ae-Ea/RT Ea/RT = 50000/(8.314 x 300) = 20.05 e-Ea/RT = 1.97 x 10-9 k = Ae-Ea/RT k = 5.90 x 10-10 |
|
Example: The rate of a reaction A(g) + B(g) → C(g) + D(g) has been studied as a function of temperature between 5000 and 18000 K. The following data were obtained for the rate constant:
Calculate the activation energy for the reaction. k = Ae-Ea/RT and lnk = lnA - Ea/RT from expt 1: → ln(5.49 x 106) = lnA - Ea/ (8.314x5000) from expt 2: → ln(9.86 x 108) = lnA - Ea/ (8.314x10000) Subtract one from the other (to remove lnA) ln(9.86 x 108) - ln(5.49 x 106) = -Ea/ (8.314x10000) + Ea/ (8.314x5000) 10000[ln(9.86 x 108) - ln(5.49 x 106)] = -Ea/8.314 + 2Ea/8.314 8.314 x 10000[ln(9.86 x 108) - ln(5.49 x 106)] = Ea 8.314 x 10000 [20.709 - 15.518] = Ea 8.314 x 10000 x 5.191 = Ea 431579.74 = Ea Ea = 432 kJ |
Note: Use of the simultaneous equation method is no longer required for first examinations 2025
Worked examples
Q641-01 The first-order reaction: 2N2O(g) → 2N2(g) + O2(g), Has a rate constant of 1.3 x 10-11 s-1 at 270°C, and 4.5 x 10-10 s-1 at 350°C. What is the activation energy for this reaction?Answer
|
k = Ae-Ea/RT and lnk = lnA - Ea/RT ln(1.3 x 10-11) = lnA - Ea/(8.314 x 543) and ln(4.5 x 10-10) = lnA - Ea/(8.314 x 623) Subtract one from the other (to remove the terms lnA) ln(4.5 x 10-10) - ln(1.3 x 10-11) = - Ea/(8.314 x 623) +
Ea/(8.314 x 543) 8.314[ln(4.5 x 10-10) - ln(1.3 x 10-11)] = (623Ea - 543Ea)/ (623 x 543) 8.314 x 623 x 543 [ln(4.5 x 10-10) - ln(1.3 x 10-11)] = 80Ea 2812534.7[-21.52 + 25.07] = 80Ea (2812534.7 x 3.5449)/80 = Ea Ea = 124806 Ea = 124.8kJ mol-1 |
Q641-02 What is the activation energy for a reaction, if its rate doubles when the temperature is raised from 20°C to 35°C?
- 342 kJ mol-1
- 269 kJ mol-1
- 34.7 kJ mol-1
- 15.1 kJ mol-1
|
If the rate doubles the rate constant doubles
when T = 293K the value for k is half that for T = 308K thus: Ae-Ea/308R = 2 x Ae-Ea/293R lnA - Ea/308R = ln2 + lnA -Ea/293R rearrange ln2 = Ea/293R - Ea/308R ln2 x 8.314 = Ea/293 - Ea/308 ln2 x 8.314 x 308 x 293 = 15Ea 519950 = 15 Ea Ea = 34663J Ea = 34.7kJ |
Q641-03 Two reactions with different activation energies have the same rate at room temperature. Which statement correctly describes the rates of these two reactions at the same higher temperature?
- The reaction with the greater activation energy will be faster.
- The reaction with the smaller activation energy will be faster.
- The two reactions will have the same rates.
- A prediction cannot be made without further information.
|
The question is asking you to consider the effect of changing T on the rate constant in the Arrhenius equation. By considering the Maxwell - Boltzmann distribution:
Increasing the temperature has a greater effect on reactions with lower activation energy Shown mathematically: k1 = A1e-Ea1/RT k2 = A2e-Ea2/RT The term e-Ea/RT has a smaller value when Ea/RT is larger For different values of Ea, the smaller Ea will give the smaller term Ea/RT for any given temperature and hence a larger value for the term e-Ea/RT. This in turn provides a higher value for the rate constant. |
Q641-04 The rate of an elementary reaction: A(g) + B(g) → C(g) + D(g) has been studied as a function of temperature between 5000 and 18000 K. The following data were obtained for the rate constant:
|
T (K)
|
k (mol dm-3/sec)
|
|
5000
|
5.49 x 106
|
|
10000
|
9.86 x 108
|
|
15000
|
5.57 x 109
|
|
18000
|
9.92 x 109
|
Calculate the activation energy for the reaction.
- 25.9 kJ/mol
- 52.0 kJ/mol
- 359 kJ/mol
- 432 kJ/mol
|
Using Arrhenius k = Ae-Ea/RT For reaction 1: 5.49 x 106 = Ae-Ea/5000R, therefore A = 5.49 x 106 /e-Ea/5000R For reaction 2: 9.86 x 108 = Ae-Ea/10000R, therefore A = 9.86 x 108 / e-Ea/10000R As A is a constant, 5.49 x 106 /e-Ea/5000R = 9.86 x 108 / e-Ea/10000R Rearrange: (5.49 x 106)/(9.86 x 108) = (e-Ea/5000R)/(e-Ea/10000R) Take natural logs: -5.19072 = (-Ea/5000R) - (-Ea/10000R) Rearrange: -5.19072 = -Ea/10000R Rearrange: Ea = 431557 = 432 kJ |
Q641-05 What happens to the rate constant (k) and the activation energy (Ea) of a reaction when the temperature is increased?
- k increases and Ea is unaffected
- k decreases and Ea is unaffected
- Ea increases and k is unaffected
- Ea decreases and k is unaffected
|
Increasing temperature has no effect on the activation energy, but it does increase the rate constant. Therefore k increases and Ea is unaffected |
Q641-06 For a given reaction in which the activation energies of the forward and reverse reactions are equal then:
- the equilibrium constant must equal one.
- the rate law can be determined from the stoichiometric equation.
- the overall order must be zero.
- ΔH must equal zero.
|
If the forward and back activation energies are equal and the value is equal to the difference between the energy level of the reactants (or products) and the highest energy state of the reaction pathway, then the enthalpy of reactants must equal the enthalpy of products. This is probably easier to see on a reaction profile graph.
|
Q641-07 To what does 'A' in the Arrhenius equation k = Ae-Ea/RT refer?
- Activation energy
- Rate constant
- Gas constant
- Collision geometry
|
The constant 'A' in the Arrhenius equation is the orientation factor, or collision geometry, a number from 0 to 1 representing the probablility of a collision being successful (assuming sufficient energy). |
Q641-08 Values of a rate constant, k, and absolute temperature, T, can be used to determine the activation energy of a reaction by a graphical method. Which graph produces a straight line?
- k versus T
- k versus 1/T
- ln k versus T
- ln k versus 1/T
|
Using the log form of the Arrhenius equation ln k = ln A -Ea/RT, and plotting ln k against 1/T gives a graph of the form y = mx + c. This is a straight line graph of gradient = -Ea/R and y intercept = ln A. |
Q641-09 A student carried out a series of experiments to find the rate constant, k, for a reaction at 300K, 400K, 500K and 600K. He plotted the following graph as data processing. Using the graph, which of the following is the most probable activation energy for this reaction?
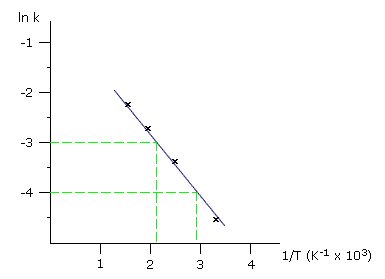
- 5.15 kJ mol-1
- 1.51 kJ mol-1
- 11.5 kJ mol-1
- 55.1 kJ mol-1
|
From the graph of ln k against 1/T gives a gradient = -Ea/R. The gradient can be estimated to equal approximately 1/0.0007 = -1250 Therefore Ea = 1250 x 8.314 = approximately 10 kJ Therefore the most likely answer is 11.5 kJ mol-1 |
Q641-10 What is the activation energy (in kJ) of a reaction whose rate constant increases by a factor of 100 upon increasing the temperature from 300 K to 360 K?
- 27
- 35
- 42
- 53
- 69
|
Using the Arrhenius equation, k = Ae-Ea/RT If k(at 360K) = 100 x k(at 300K), then Ae-Ea/RT(at 360K) = 100 x Ae-Ea/RT(at 300K) Take log base e: ln A - Ea/360R = ln 100 + ln A - Ea/300R Ea/300R - Ea/360R = ln100 = 4.605 Multiply through by 300R: Ea - 5Ea/6 = 4.605 x 300 x 8.314 Multiply through by 6: 6Ea - 5Ea = 68915 J Therefore Ea = 68.9 kJ |